Understanding The Concept Of Subtracting Squares From Squares

Subtracting squares from squares is a fascinating concept that bridges the gap between basic arithmetic and advanced mathematical thinking. This intriguing topic explores the subtraction of one perfect square from another, uncovering patterns, relationships, and properties that inspire curiosity and problem-solving. Whether you're a student, educator, or math enthusiast, diving into this subject offers a rewarding way to deepen your understanding of mathematics.

The beauty of subtracting squares from squares lies in its versatility and applications. From algebraic identities to geometric interpretations, this mathematical concept is more than just a calculation—it's a gateway to uncovering the underlying structure of numbers. By exploring this topic, you'll not only sharpen your mathematical skills but also gain insights into how seemingly simple operations can lead to profound discoveries.

If you're wondering why subtracting squares from squares matters, consider its role in problem-solving, number theory, and even real-world applications. This article will guide you through the principles, techniques, and examples of this concept, ensuring you have everything you need to master it. Let's jump into this mathematical journey and unravel the mysteries of subtracting squares from squares!

Table of Contents

• What is Subtracting Squares from Squares?
• Why is Subtracting Squares from Squares Important?
• The Mathematical Formula for Subtracting Squares
• How to Solve Problems Using Subtracting Squares from Squares?
• Geometric Interpretation of Subtracting Squares
• Real-World Applications of Subtracting Squares from Squares
• Common Mistakes and How to Avoid Them?
• Examples of Subtracting Squares from Squares
• Exploring Patterns in Subtracting Squares from Squares
• Advanced Techniques for Subtracting Squares
• How Does Subtracting Squares Relate to Algebra?
• What are the Challenges in Subtracting Squares?
• History of Subtracting Squares from Squares
• Tips for Mastering Subtracting Squares from Squares
• Conclusion

What is Subtracting Squares from Squares?

At its core, subtracting squares from squares involves taking one perfect square number and subtracting another perfect square number from it. For example, if you have two squares, such as \(9\) (which is \(3^2\)) and \(4\) (which is \(2^2\)), subtracting them gives \(9 - 4 = 5\). This operation often reveals unique properties of numbers, such as differences of squares or interesting patterns in arithmetic.

Why is Subtracting Squares from Squares Important?

Understanding subtracting squares from squares is essential because it lays the groundwork for more advanced mathematical concepts. It’s a fundamental topic in algebra, number theory, and geometry. Additionally, this concept has real-world applications, such as solving optimization problems, modeling physical phenomena, and even cryptographic systems.

The Mathematical Formula for Subtracting Squares

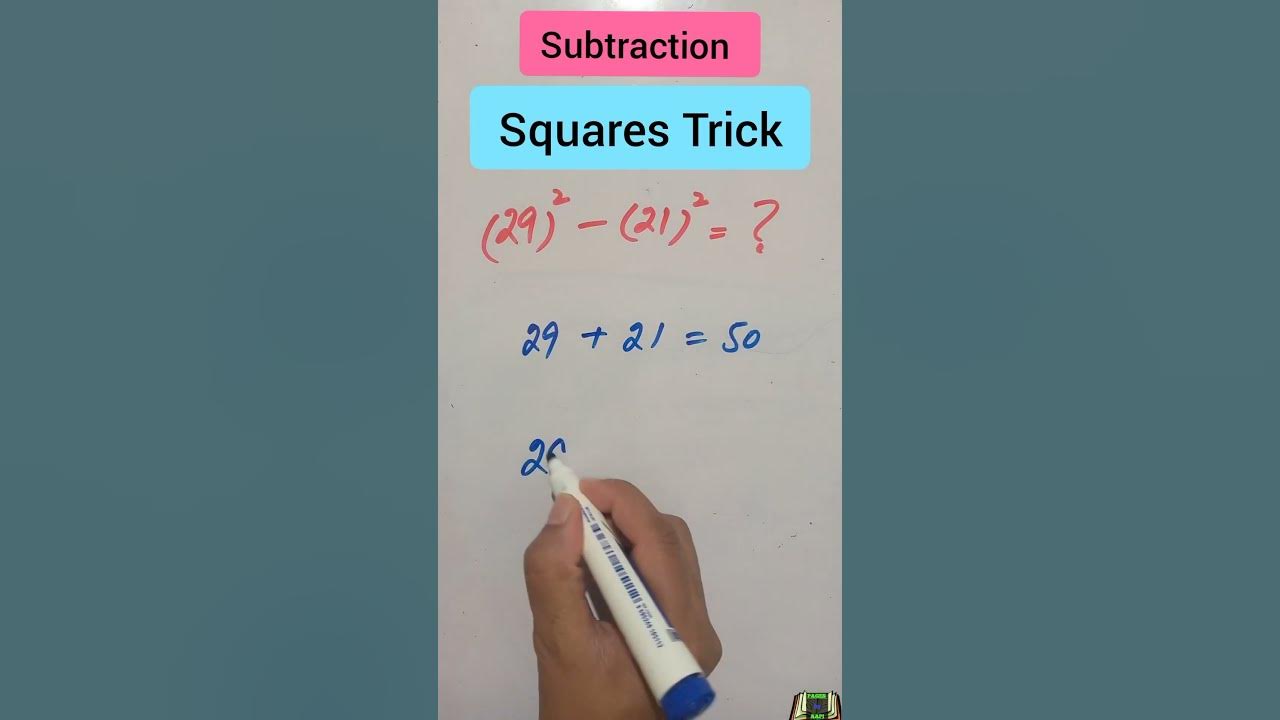
One of the most well-known formulas related to subtracting squares is the difference of squares identity: \(a^2 - b^2 = (a - b)(a + b)\). This formula allows you to factorize the result of subtracting two squares efficiently, making it a valuable tool in algebraic problem-solving.

How Does the Formula Work?

The difference of squares formula works by breaking down the subtraction of two square terms into the product of two binomials. For example, if you have \(16 - 9\), you can rewrite it as \((4 - 3)(4 + 3) = 7 \times 1 = 7\).

How to Solve Problems Using Subtracting Squares from Squares?

To solve problems involving subtracting squares from squares, follow these steps:

1. Identify the two square numbers involved.
2. Apply the difference of squares formula, if applicable.
3. Simplify the expression to find the result.
4. Verify your answer by reversing the operation.

Example Problem

Find the result of \(25 - 16\). Using the formula \(a^2 - b^2 = (a - b)(a + b)\), we can rewrite it as \((5 - 4)(5 + 4) = 1 \times 9 = 9\).

Geometric Interpretation of Subtracting Squares

Subtracting squares from squares can also be visualized geometrically. Imagine two squares of different sizes. If you remove the area of the smaller square from the larger square, the remaining area represents the difference between the two squares. This visualization helps in understanding the concept intuitively.

Real-World Applications of Subtracting Squares from Squares

Beyond theoretical mathematics, subtracting squares from squares appears in various real-world scenarios:

• Designing geometric shapes and patterns
• Calculating areas in architecture and engineering
• Optimizing algorithms in computer science
• Modeling problems in physics and economics

Common Mistakes and How to Avoid Them?

When working with subtracting squares from squares, some common mistakes include:

• Forgetting to square the numbers initially
• Incorrectly applying the difference of squares formula
• Overlooking negative results when subtracting larger numbers from smaller ones

To avoid these errors, double-check your calculations and ensure you understand the underlying principles before attempting complex problems.

Examples of Subtracting Squares from Squares

Here are a few examples to practice and understand this concept:

1. \(49 - 36 = (7 - 6)(7 + 6) = 1 \times 13 = 13\)
2. \(64 - 25 = (8 - 5)(8 + 5) = 3 \times 13 = 39\)
3. \(100 - 81 = (10 - 9)(10 + 9) = 1 \times 19 = 19\)

Exploring Patterns in Subtracting Squares from Squares

One interesting aspect of subtracting squares from squares is the patterns that emerge. For instance, the results are often odd numbers, and the difference between consecutive squares follows an arithmetic progression. Exploring these patterns can lead to deeper insights into number theory.

Advanced Techniques for Subtracting Squares

For more complex problems, advanced techniques such as modular arithmetic, algebraic manipulation, and calculus can be employed. These approaches allow for solving problems involving larger numbers or multiple variables.

How Does Subtracting Squares Relate to Algebra?

Subtracting squares from squares is closely tied to algebraic concepts such as factorization, equations, and inequalities. The difference of squares formula, in particular, is a cornerstone of algebra that simplifies expressions and solves equations efficiently.

What are the Challenges in Subtracting Squares?

Some challenges in subtracting squares from squares include dealing with negative results, working with non-integer values, and applying the concept to abstract problems. Overcoming these challenges requires a solid understanding of the fundamentals and practice with diverse problem sets.

History of Subtracting Squares from Squares

The concept of subtracting squares from squares has roots in ancient mathematics, with evidence of its use in Greek geometry and Indian algebra. Over centuries, this principle has evolved into a foundational topic in modern mathematics, influencing various fields of study.

Tips for Mastering Subtracting Squares from Squares

Here are some tips to help you master subtracting squares from squares:

• Practice regularly with a variety of problems
• Visualize the concept geometrically
• Learn and apply the difference of squares formula
• Explore advanced techniques and applications

Conclusion

Subtracting squares from squares is more than just a mathematical operation—it's a gateway to understanding deeper principles and patterns in mathematics. By exploring this topic, you can enhance your problem-solving skills, uncover fascinating connections between numbers, and apply these insights to real-world scenarios. Whether you're a beginner or an advanced learner, the journey into subtracting squares from squares offers endless opportunities for discovery and growth.