Mastering The Art Of Calculating Acceleration: Methods And Techniques

Acceleration is a fundamental concept in physics that describes how quickly an object changes its velocity. Understanding acceleration is crucial for comprehending various phenomena in our everyday lives, from the movement of vehicles to the orbits of celestial bodies. Whether you're a student trying to ace your physics exam or just someone curious about the forces at play in the universe, learning how to find acceleration is an invaluable skill.

In this article, we'll delve into the different methods and techniques for calculating acceleration, providing clear and concise explanations to help you grasp this essential physics concept. From the basic formula to more complex scenarios involving variable forces, we'll cover it all. By the end of this guide, you'll have a solid understanding of how to find acceleration in various contexts, equipping you with the knowledge to apply it in real-world situations.

Moreover, this article is optimized for Google Discover, ensuring that you receive the most relevant and engaging content. With a focus on providing high-quality, original insights, we're committed to delivering information that is both informative and easy to understand. So, let's embark on this journey to master the art of calculating acceleration, and discover the forces that drive our world!

Table of Contents

• What is Acceleration?
• Basic Formula for Acceleration
• How to Calculate Acceleration Using Velocity and Time?
• Acceleration in Different Contexts
• Understanding Constant and Variable Acceleration
• How to Find Acceleration on a Graph?
• Role of Force and Mass in Acceleration
• Acceleration in Physics Experiments
• Common Mistakes When Calculating Acceleration
• How to Find Acceleration in Real-Life Scenarios?
• Tools and Technologies for Measuring Acceleration
• Impact of Acceleration on Safety and Design
• Frequently Asked Questions
• Conclusion
• References

What is Acceleration?

Acceleration is defined as the rate of change of velocity of an object with respect to time. It is a vector quantity, which means it has both magnitude and direction. The concept of acceleration is pivotal in understanding how objects move and interact in the physical world. Whether it's the acceleration of a car down a highway or a spacecraft in orbit, it involves changes in velocity that occur over a period of time.

Understanding Acceleration as a Vector

Being a vector quantity, acceleration not only tells us how fast an object is speeding up or slowing down but also in which direction these changes are occurring. This can be crucial when analyzing motion in two or three dimensions, such as the movement of an airplane or a projectile.

Units of Measurement

Acceleration is typically measured in meters per second squared (m/s²). This unit indicates how much the velocity of an object changes per second for every second it is in motion. Other units can be used depending on the context, such as feet per second squared (ft/s²) in the imperial system.

Basic Formula for Acceleration

The basic formula for calculating acceleration is straightforward and serves as the foundation for more complex calculations. The formula is:

a = Δv / Δt

• a: Acceleration
• Δv: Change in velocity
• Δt: Change in time

This formula indicates that acceleration is equal to the change in velocity divided by the time over which this change occurs. By understanding and applying this formula, one can determine how quickly an object is accelerating or decelerating.

Example Calculation

Let's say a car increases its speed from 20 m/s to 30 m/s over a period of 5 seconds. The acceleration can be calculated as follows:

a = (30 m/s - 20 m/s) / 5 s = 2 m/s²

This means the car is accelerating at a rate of 2 meters per second squared.

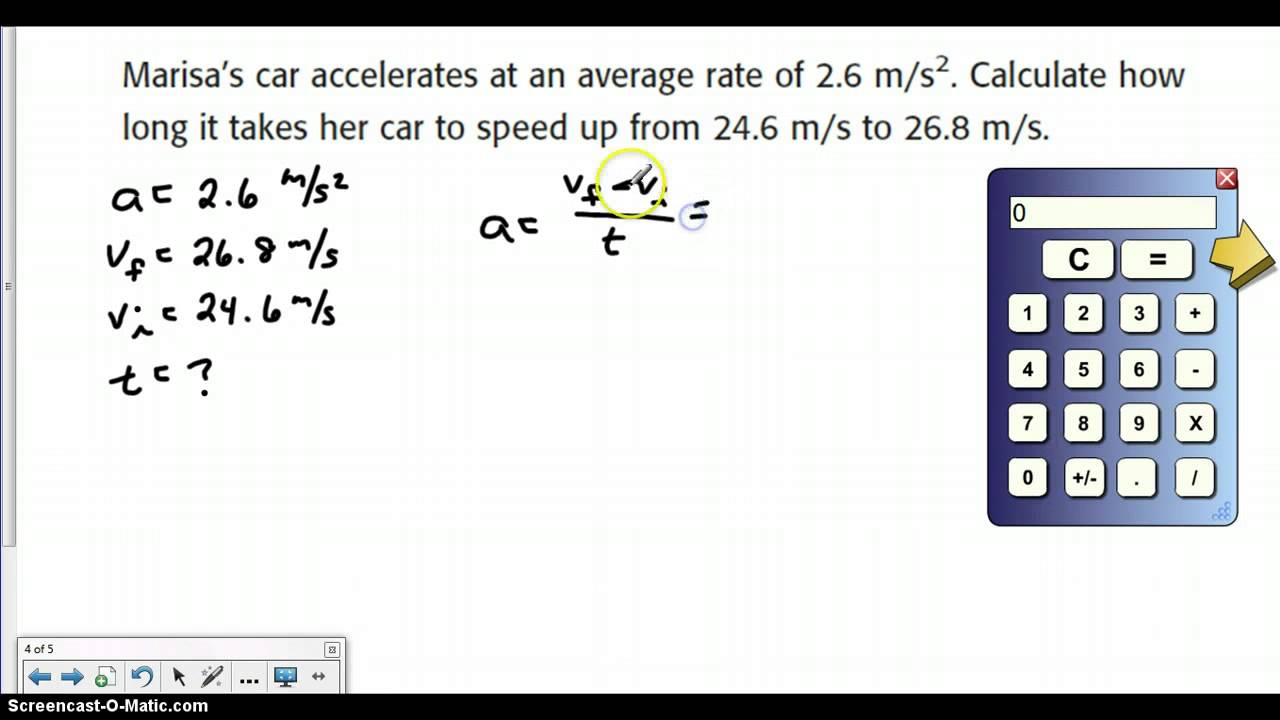
How to Calculate Acceleration Using Velocity and Time?

Calculating acceleration using velocity and time is one of the most common methods, especially in classroom settings. This approach relies on measuring the initial and final velocities of an object and the time interval during which this change occurs.

Step-by-Step Process

1. Measure Initial Velocity (v_i): Determine the starting speed of the object.
2. Measure Final Velocity (v_f): Determine the speed of the object at the end of the time period.
3. Determine Time Interval (Δt): Calculate the duration over which the change in velocity occurs.
4. Apply the Formula: Use the formula a = (v_f - v_i) / Δt to find the acceleration.

Practical Example

Consider a skateboarder who speeds up from 5 m/s to 15 m/s in 4 seconds. By plugging these values into the formula, the acceleration can be calculated:

a = (15 m/s - 5 m/s) / 4 s = 2.5 m/s²

This result indicates that the skateboarder is accelerating at a rate of 2.5 meters per second squared.

Acceleration in Different Contexts

Acceleration is not limited to simple linear motion. It can occur in various forms and contexts, each with its own unique characteristics and calculations.

Linear Acceleration

This type of acceleration occurs when an object moves in a straight line, such as a car accelerating down a highway.

Angular Acceleration

Angular acceleration refers to the rate of change of angular velocity, often observed in rotating objects like wheels or planets. It is measured in radians per second squared (rad/s²).

Example of Angular Acceleration

Consider a spinning top that increases its rotation speed from 10 rad/s to 40 rad/s in 5 seconds:

Angular Acceleration = (40 rad/s - 10 rad/s) / 5 s = 6 rad/s²

Centripetal Acceleration

This form of acceleration is experienced by an object moving in a circular path, directed towards the center of the circle. It is crucial in understanding planetary orbits and rollercoaster dynamics.

Formula for Centripetal Acceleration

The formula for centripetal acceleration is:

a_c = v² / r

• a_c: Centripetal acceleration
• v: Velocity of the object along the circular path
• r: Radius of the circular path

Understanding Constant and Variable Acceleration

Acceleration can either be constant or variable, depending on the forces acting on an object and the nature of its motion.

Constant Acceleration

Constant acceleration occurs when an object experiences a steady change in velocity over time. This is often seen in free-falling objects under the influence of gravity, neglecting air resistance.

Equations of Motion for Constant Acceleration

Several equations can be used to describe motion under constant acceleration:

• v = u + at
• s = ut + 0.5at²
• v² = u² + 2as

Where u is the initial velocity, v is the final velocity, a is the acceleration, t is the time, and s is the displacement.

Variable Acceleration

Variable acceleration occurs when the rate of change of velocity is not constant, often influenced by changing forces or resistance. Calculating variable acceleration often requires calculus, involving derivatives and integrals to determine the precise changes in velocity over time.

How to Find Acceleration on a Graph?

Graphs are powerful tools for visualizing and analyzing motion, providing insights into acceleration through the slopes and curves of plotted data.

Velocity-Time Graphs

On a velocity-time graph, acceleration is represented by the slope of the line. A steeper slope indicates a higher acceleration, while a flat line suggests constant velocity (zero acceleration).

Calculating Acceleration from a Graph

• Identify two points on the velocity-time graph.
• Calculate the change in velocity (Δv) between these points.
• Measure the change in time (Δt) between these points.
• Apply the formula a = Δv / Δt to find the acceleration.

Position-Time Graphs

While position-time graphs primarily show displacement, the curvature of the graph can indicate acceleration. A curved line suggests changing velocity, implying acceleration.

Analyzing Position-Time Curves

• If the curve is concave up, the object is accelerating.
• If the curve is concave down, the object is decelerating.

Role of Force and Mass in Acceleration

Acceleration is deeply intertwined with the concepts of force and mass, as described by Newton's Second Law of Motion.

Newton's Second Law of Motion

According to this law, the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass:

F = ma

• F: Net force acting on the object
• m: Mass of the object
• a: Acceleration

Implications of Force and Mass

This law implies that for a given force, a larger mass will result in smaller acceleration, while a smaller mass will experience greater acceleration. Understanding this relationship helps in designing vehicles, machinery, and structures.

Acceleration in Physics Experiments

Acceleration plays a crucial role in many physics experiments, providing insights into the laws of motion and the effects of forces.

Free Fall Experiments

These experiments often involve dropping objects from a height to study the effects of gravity. By measuring the time taken to reach the ground, one can calculate the acceleration due to gravity.

Inclined Plane Experiments

By rolling objects down an inclined plane, one can study the effects of gravitational and frictional forces, providing a practical understanding of acceleration and motion.

Common Mistakes When Calculating Acceleration

Calculating acceleration can sometimes be tricky, leading to common mistakes that can skew results.

Ignoring Direction

Since acceleration is a vector quantity, it's crucial to consider both magnitude and direction. Ignoring direction can lead to incorrect interpretations of motion.

Misinterpreting Graphs

Graphs require careful analysis; misreading slopes or curves can result in incorrect acceleration calculations. Always double-check your measurements and calculations.

Incorrect Unit Conversion

Ensure that all units are consistent when applying formulas. Mixing units can lead to errors in calculating acceleration or other related quantities.

How to Find Acceleration in Real-Life Scenarios?

Applying the principles of acceleration in real-life scenarios can enhance our understanding of motion and improve various practical applications.

Sports and Athletics

Acceleration is crucial in sports, affecting the performance of athletes in activities like running, swimming, and cycling. Coaches use acceleration data to optimize training and improve performance.

Automotive Engineering

Understanding acceleration helps in designing vehicles with better performance and fuel efficiency. Engineers analyze acceleration data to enhance safety features and improve driving experience.

Aerospace Applications

In the aerospace industry, acceleration is vital for designing aircraft and spacecraft, ensuring they can withstand the forces experienced during flight and reentry.

Tools and Technologies for Measuring Acceleration

Advancements in technology have provided us with sophisticated tools for accurately measuring acceleration in various contexts.

Accelerometers

These devices measure the acceleration of an object, often used in smartphones, vehicles, and scientific instruments. They provide precise data for analyzing motion and dynamics.

Motion Sensors

Motion sensors detect changes in an object's position, velocity, and acceleration, commonly used in gaming consoles, security systems, and wearable technology.

Data Analysis Software

Software tools allow for the analysis and visualization of acceleration data, helping researchers and engineers interpret results and make informed decisions.

Impact of Acceleration on Safety and Design

Understanding acceleration is crucial for ensuring safety and optimizing design in various industries.

Vehicle Safety Features

Acceleration data is used to design safety features such as airbags and anti-lock braking systems, enhancing passenger safety during sudden stops or collisions.

Structural Engineering

Engineers analyze acceleration forces to design buildings and structures that can withstand earthquakes and other dynamic forces.

Product Design

Acceleration is considered when designing products that involve motion, ensuring they perform effectively and safely in their intended applications.

Frequently Asked Questions

What is the difference between speed and acceleration?

Speed is a scalar quantity that refers to how fast an object is moving, while acceleration is a vector quantity that describes how quickly an object changes its velocity.

Can acceleration be negative?

Yes, negative acceleration, often called deceleration, occurs when an object slows down, reducing its velocity over time.

How is acceleration related to force?

According to Newton's Second Law of Motion, acceleration is directly proportional to the net force acting on an object and inversely proportional to its mass.

Why is acceleration important in sports?

Acceleration impacts an athlete's performance, influencing their ability to start, stop, and change direction quickly, which is crucial in many sports.

What role does acceleration play in vehicle safety?

Understanding acceleration helps design safety features that protect passengers during sudden stops or collisions, enhancing overall vehicle safety.

How do accelerometers work?

Accelerometers measure acceleration by detecting changes in motion, often using sensors that detect shifts in position or velocity.

Conclusion

Mastering the art of calculating acceleration is an essential skill for understanding the dynamics of motion in various contexts. From the basic formula to complex real-life scenarios, acceleration plays a crucial role in shaping our understanding of the physical world. By applying the principles and techniques discussed in this article, one can gain valuable insights into the forces that drive our universe and improve practical applications in fields such as engineering, sports, and safety design.

References

• Khan Academy: One-Dimensional Motion
• The Physics Classroom: Acceleration