Complex Numbers: A Deep Dive Into Their Significance And Applications

Complex numbers are a fascinating aspect of mathematics that extend beyond the realms of real numbers. They comprise a real part and an imaginary part, providing a comprehensive way to solve equations that have no real solutions. Originating from the need to find the square roots of negative numbers, complex numbers have become a fundamental part of various mathematical fields, including algebra, calculus, and even quantum mechanics. This article will explore the myriad of ways complex numbers are utilized and their significance in both theoretical and applied mathematics.

The concept of complex numbers can appear daunting at first, but when broken down, they offer a unique perspective on problem-solving. By incorporating both real and imaginary numbers, complex numbers allow for a broader range of solutions and insights into mathematical problems. They are represented in the form of a + bi, where 'a' is the real part and 'bi' is the imaginary part, with 'i' denoting the imaginary unit. This form not only facilitates the manipulation of mathematical equations but also enhances our understanding of the mathematical universe.

As we delve into the world of complex numbers, it becomes evident that their applications are far-reaching. From electrical engineering to fluid dynamics, complex numbers play a crucial role in modeling and solving real-world problems. Their ability to simplify calculations and provide solutions where none previously existed highlights their importance in modern science and engineering. This article aims to provide an in-depth exploration of complex numbers, their history, properties, and the various ways they have revolutionized mathematical thinking.

Table of Contents

• History of Complex Numbers
• What Are Complex Numbers?
• The Significance of the Imaginary Unit
• Properties of Complex Numbers
• How to Represent Complex Numbers?
• Applications in Electrical Engineering
• Complex Numbers in Fluid Dynamics
• Complex Numbers and Quantum Mechanics
• Role in Algebra and Calculus
• Visualizing Complex Numbers
• Complex Conjugates and Their Importance
• Polar Form of Complex Numbers
• Frequently Asked Questions
• Conclusion
• External Links and References

History of Complex Numbers

The journey of complex numbers began with attempts to solve polynomial equations that had no real solutions. The earliest recorded use of complex numbers dates back to the 16th century when Italian mathematician Gerolamo Cardano encountered the square roots of negative numbers while solving cubic equations. However, it wasn't until the 18th century that complex numbers gained recognition, thanks to the contributions of mathematicians like Euler and Gauss.

Euler's introduction of the imaginary unit 'i' as the square root of -1 and his famous formula, e^(iπ) + 1 = 0, laid the groundwork for complex analysis. Gauss further expanded on this by introducing the complex plane, a graphical representation of complex numbers. This breakthrough enabled mathematicians to visualize and manipulate complex numbers more effectively, leading to advancements in numerous mathematical disciplines.

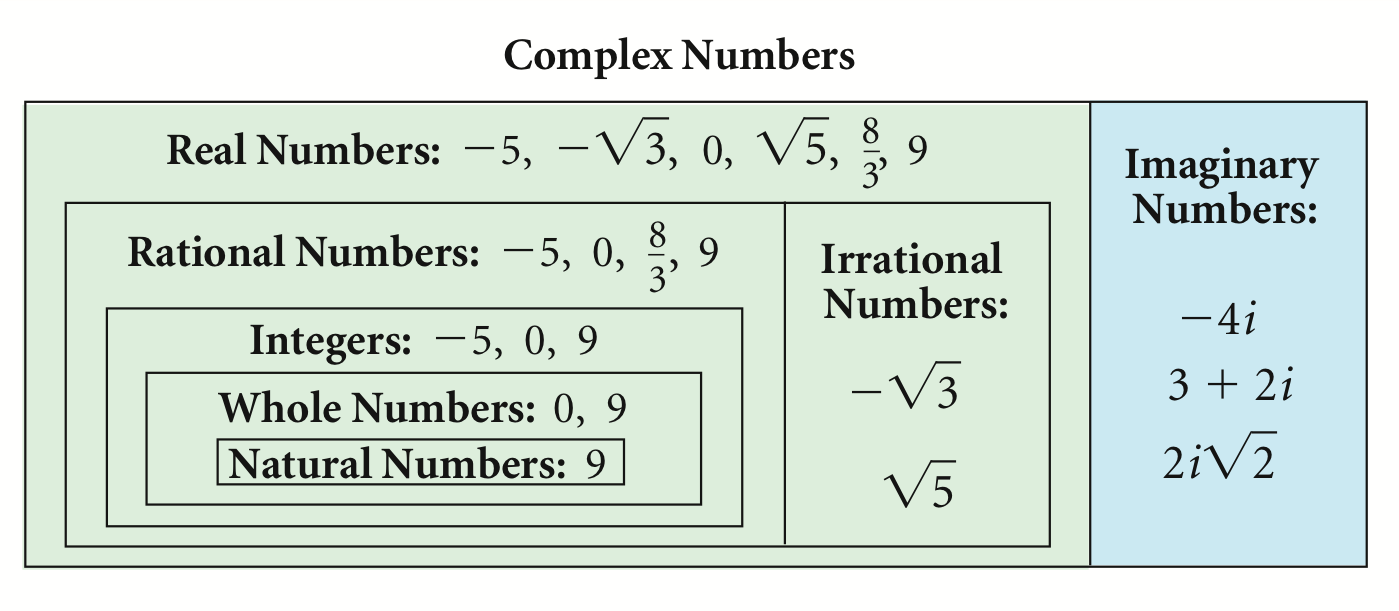
What Are Complex Numbers?

Complex numbers are numbers that consist of a real part and an imaginary part. They can be expressed in the form a + bi, where 'a' represents the real component, and 'bi' signifies the imaginary component. In this notation, 'b' is a real number, and 'i' is the imaginary unit defined by the property i² = -1.

The introduction of complex numbers allows for solutions to equations that would otherwise be unsolvable within the domain of real numbers. For example, the equation x² + 1 = 0 has no real solutions, but it has complex solutions, x = i and x = -i. This ability to extend the number system provides a more comprehensive framework for mathematical analysis and problem-solving.

The Significance of the Imaginary Unit

The imaginary unit 'i' is the cornerstone of complex numbers. Defined as the square root of -1, it allows for the creation of numbers that do not exist on the real number line. The imaginary unit is crucial for understanding complex numbers, as it facilitates the representation and manipulation of these numbers in mathematical equations and calculations.

The concept of imaginary numbers might initially seem abstract, but they have practical applications in various fields. For instance, in electrical engineering, the imaginary unit is used to represent and analyze alternating current (AC) circuits. By using complex numbers, engineers can simplify complex calculations and gain insights into circuit behavior that would be challenging to achieve with real numbers alone.

Properties of Complex Numbers

Complex numbers have several unique properties that distinguish them from real numbers. These properties include:

• Addition and Subtraction: Complex numbers are added and subtracted by combining their real parts and imaginary parts separately.
• Multiplication: The multiplication of complex numbers involves distributing and using the property i² = -1 to simplify the result.
• Conjugate: The conjugate of a complex number a + bi is a - bi. Conjugates are used in division and simplifying complex expressions.
• Modulus: The modulus of a complex number is its distance from the origin in the complex plane, calculated as √(a² + b²).
• Argument: The argument of a complex number is the angle it forms with the positive real axis, measured in radians or degrees.

How to Represent Complex Numbers?

Complex numbers can be represented in two primary forms: rectangular form and polar form. The rectangular form is the standard representation a + bi, where 'a' is the real part and 'bi' is the imaginary part. This form is advantageous for addition, subtraction, and direct calculations.

The polar form, on the other hand, represents complex numbers in terms of their modulus and argument. It is expressed as r(cos θ + i sin θ) or re^(iθ), where 'r' is the modulus, and 'θ' is the argument. The polar form is particularly useful for multiplication and division, as it simplifies the process by converting complex arithmetic into operations on magnitudes and angles.

Applications in Electrical Engineering

Complex numbers are indispensable in electrical engineering, particularly in the analysis of AC circuits. They are used to represent impedances, voltages, and currents in a concise manner, facilitating calculations and circuit analysis. By using complex numbers, engineers can simplify the process of solving circuit equations and gain valuable insights into the behavior of electrical systems.

How do complex numbers simplify circuit analysis?

The use of complex numbers in circuit analysis allows for the representation of sinusoidal waveforms as phasors. Phasors are complex numbers that encapsulate both the magnitude and phase of a sinusoidal function. This representation simplifies the analysis of AC circuits by converting differential equations into algebraic equations, making the solution process more manageable.

What are phasors in electrical engineering?

Phasors are a mathematical representation of sinusoidal waveforms in terms of complex numbers. They are used to describe the amplitude and phase angle of AC signals, enabling engineers to analyze and visualize the behavior of electrical circuits. By using phasors, engineers can perform operations such as addition, subtraction, and multiplication on sinusoidal signals, simplifying the analysis of complex electrical systems.

Complex Numbers in Fluid Dynamics

In fluid dynamics, complex numbers are used to model and analyze fluid flow patterns and behavior. They are particularly useful in solving problems related to potential flow, where the complex potential function is used to represent the flow of an ideal fluid.

The complex potential function combines the velocity potential and stream function into a single complex function. This allows for the visualization and analysis of fluid flow patterns, such as the flow around objects and the behavior of vortices. By using complex numbers, fluid dynamicists can simplify the mathematical representation of fluid flow and gain insights into the underlying physics.

Complex Numbers and Quantum Mechanics

Complex numbers play a crucial role in quantum mechanics, where they are used to represent wave functions and probability amplitudes. Quantum mechanics relies heavily on complex numbers to describe the behavior of particles at the quantum level, as they provide a comprehensive framework for understanding phenomena that cannot be explained using real numbers alone.

In quantum mechanics, the wave function is a complex-valued function that encodes the probabilities of finding a particle in different states. The use of complex numbers allows for the representation of superposition and interference, which are fundamental concepts in quantum mechanics. By employing complex numbers, physicists can accurately model and predict the behavior of quantum systems.

Role in Algebra and Calculus

Complex numbers have a significant impact on algebra and calculus, enabling the solution of equations and the analysis of functions that involve imaginary components. They extend the real number system, allowing for a more comprehensive understanding of mathematical relationships and operations.

In algebra, complex numbers are used to solve polynomial equations that have no real solutions. They provide a complete set of solutions and enable the factorization of polynomials over the complex field. In calculus, complex numbers are used in complex analysis, a branch of mathematics that studies functions of complex variables. Complex analysis has applications in various fields, including fluid dynamics, electromagnetism, and signal processing.

Visualizing Complex Numbers

The visualization of complex numbers is typically done using the complex plane, a two-dimensional plane with a real axis and an imaginary axis. Each complex number is represented as a point on this plane, with the real part corresponding to the x-coordinate and the imaginary part corresponding to the y-coordinate.

This graphical representation allows for the visualization of complex number operations, such as addition, subtraction, and multiplication. For example, the addition of two complex numbers corresponds to the vector addition of their points on the complex plane. The complex plane also provides a way to visualize the modulus and argument of complex numbers, aiding in the understanding of their properties and applications.

Complex Conjugates and Their Importance

Complex conjugates are pairs of complex numbers that have the same real part but opposite imaginary parts. The complex conjugate of a complex number a + bi is a - bi. Complex conjugates play a vital role in various mathematical operations, including division and the simplification of complex expressions.

One of the key properties of complex conjugates is that their product is a real number. This property is used to rationalize denominators in complex fractions and to simplify expressions involving complex numbers. Complex conjugates are also used in the calculation of the modulus and argument of complex numbers, providing valuable insights into their behavior and properties.

Polar Form of Complex Numbers

The polar form of complex numbers is a representation that expresses complex numbers in terms of their modulus and argument. It is given by r(cos θ + i sin θ) or re^(iθ), where 'r' is the modulus, and 'θ' is the argument. The polar form is particularly useful for multiplication and division, as it simplifies these operations by converting them into operations on magnitudes and angles.

The polar form also provides a convenient way to visualize complex numbers and their operations. By representing complex numbers as points in the polar coordinate system, it is easier to understand their behavior and properties. The polar form is widely used in engineering and physics, particularly in the analysis of oscillatory systems and waveforms.

Frequently Asked Questions

What are complex numbers used for?

Complex numbers are used in various fields, including engineering, physics, and mathematics, to solve equations and model systems that involve imaginary components. They provide a comprehensive framework for understanding and analyzing complex phenomena.

How are complex numbers represented?

Complex numbers are represented in the form a + bi, where 'a' is the real part and 'bi' is the imaginary part. They can also be represented in polar form as r(cos θ + i sin θ) or re^(iθ).

What is the significance of the imaginary unit?

The imaginary unit 'i' is defined as the square root of -1 and is crucial for representing and manipulating complex numbers. It allows for the creation of numbers that do not exist on the real number line, providing a broader range of solutions to mathematical problems.

How do complex numbers simplify circuit analysis?

Complex numbers simplify circuit analysis by representing sinusoidal waveforms as phasors, which encapsulate both the magnitude and phase of AC signals. This representation converts differential equations into algebraic equations, making the solution process more manageable.

What is the role of complex numbers in quantum mechanics?

Complex numbers are used in quantum mechanics to represent wave functions and probability amplitudes. They provide a comprehensive framework for understanding and predicting the behavior of particles at the quantum level.

How do complex conjugates simplify mathematical expressions?

Complex conjugates simplify mathematical expressions by providing a way to rationalize denominators in complex fractions and calculate the modulus and argument of complex numbers. Their product is a real number, which aids in the simplification of complex expressions.

Conclusion

Complex numbers are a fundamental aspect of mathematics that extend beyond the limitations of real numbers. Their ability to represent and solve equations with imaginary components has revolutionized various fields, including engineering, physics, and mathematics. From simplifying circuit analysis in electrical engineering to modeling fluid flow in fluid dynamics, complex numbers play a crucial role in modern science and technology.

By providing a comprehensive framework for understanding complex phenomena, complex numbers have enhanced our ability to model, analyze, and predict the behavior of systems that involve imaginary components. Their significance and applications continue to grow, making them an indispensable tool in the world of mathematics and beyond.

External Links and References

• Khan Academy: Complex Numbers
• Math is Fun: Complex Numbers
• Encyclopedia Britannica: Complex Number